Are you hoping to find 'modular arithmetic essay'? You can find your answers here.
Table of contents
- Modular arithmetic essay in 2021
- Modular arithmetic calendar
- Modular arithmetic proofs
- Application of modular arithmetic examples
- Division of modular arithmetic
- Modulo 6 addition table
- How to do modular arithmetic
- Modular arithmetic latex
Modular arithmetic essay in 2021
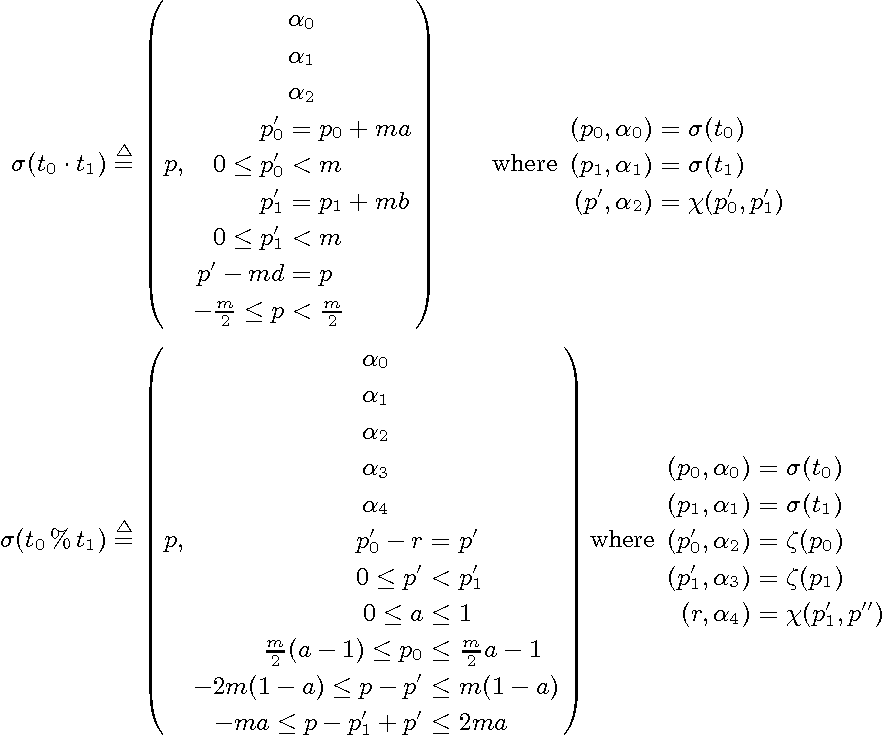 This picture illustrates modular arithmetic essay.
This picture illustrates modular arithmetic essay.
Modular arithmetic calendar
 This image representes Modular arithmetic calendar.
This image representes Modular arithmetic calendar.
Modular arithmetic proofs
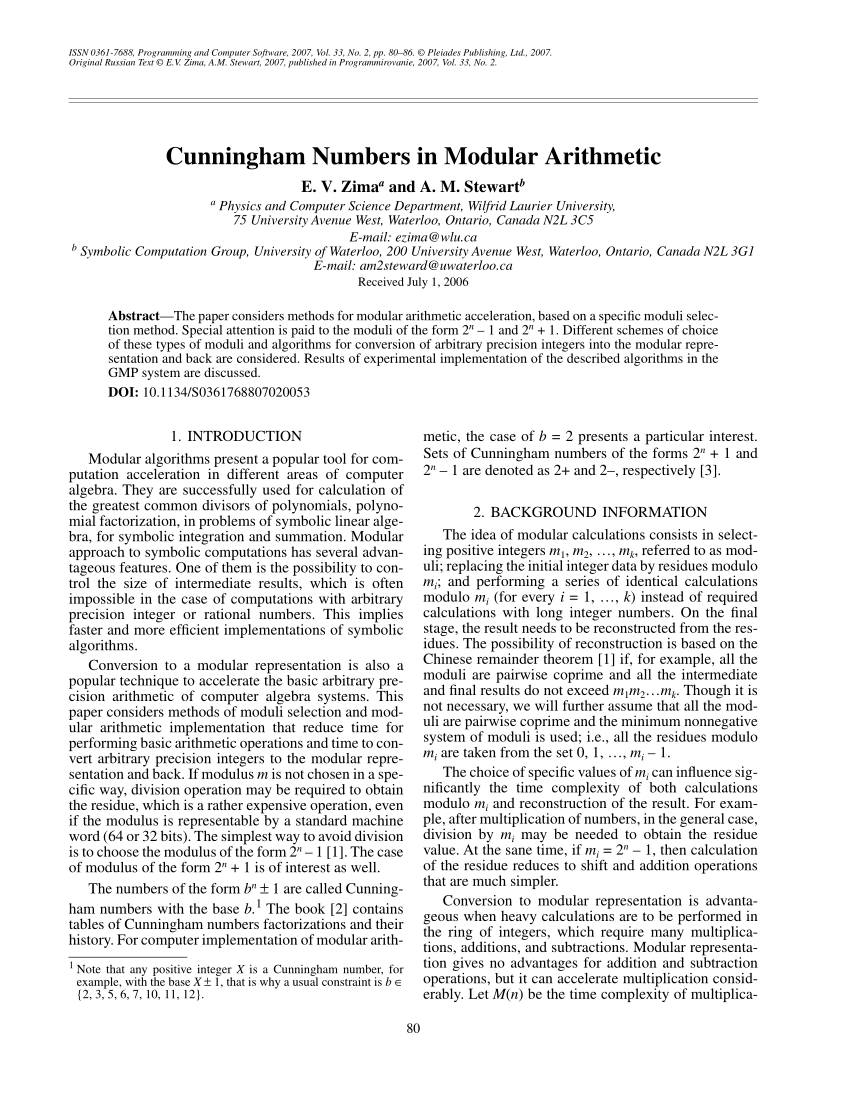 This image illustrates Modular arithmetic proofs.
This image illustrates Modular arithmetic proofs.
Application of modular arithmetic examples
 This image illustrates Application of modular arithmetic examples.
This image illustrates Application of modular arithmetic examples.
Division of modular arithmetic
 This image representes Division of modular arithmetic.
This image representes Division of modular arithmetic.
Modulo 6 addition table
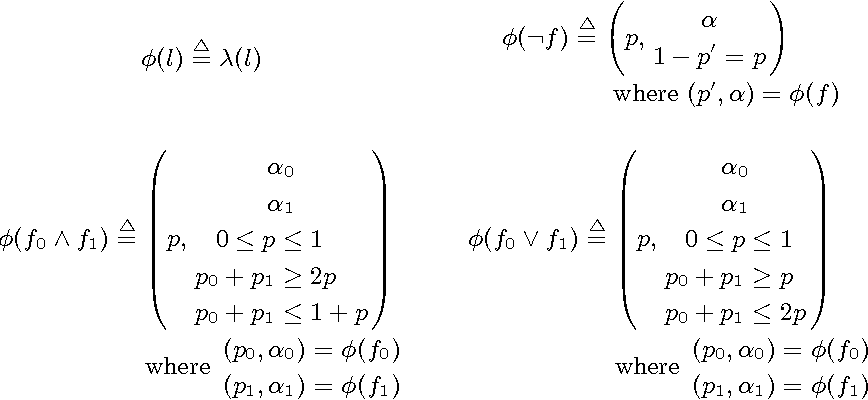 This picture shows Modulo 6 addition table.
This picture shows Modulo 6 addition table.
How to do modular arithmetic
 This picture illustrates How to do modular arithmetic.
This picture illustrates How to do modular arithmetic.
Modular arithmetic latex
 This picture demonstrates Modular arithmetic latex.
This picture demonstrates Modular arithmetic latex.
When is rmodn equal to the remainder in modular arithmetic?
As a quick review, rmodn is equal to the remainder when we divide r by n. Addition, subtraction, and multiplication in modular arithmetic obey two basic rules. If a + b = c, then (a + b)modn is congruent to cmodn. If amodn is congruent to dmodn and bmodn is congruent to emodn, then (a + b)modn is congruent to dmodn + emodn.
Which is congruent to 3mod17 in modular arithmetic?
Working in modulus 17, find (18*20)mod17. If we perform the multiplication first, we get 18*20 = 360, so (18*20)mod17 is congruent to 360mod17. The remainder when we divide 360 by 17 is 3, so 360mod17 is congruent to 3mod17. Thus, (18*20)mod17 is congruent to 3mod17.
When did Carl Friedrich Gauss introduce modular arithmetic?
The conventional arithmetic is based on linear number system known as the ‘ number line’. Modular Arithemetic was introduced by Carl Friedrich Gauss in 1801, in his book ‘ Disquisitiones Arithmeticae’. (modular). It is based on circle. A circle can be divided into any number of parts.
When do you use modular arithmetic in math?
Modular arithmetic is a useful tool in mathematics. When adding, subtracting, or multiplying in modular arithmetic, we can either first perform the operation and then find that number in the given modulus, or we can find each number in the given modulus and then perform the operation.
Last Update: Oct 2021